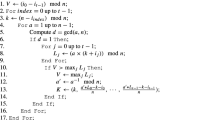Abstract
The higher order nonlinearity of a Boolean function is a cryptographic criterion, which plays an important role in the design of secure block ciphers and stream ciphers. In this paper, we obtain lower bounds of second-order nonlinearities of two classes of highly nonlinear cubic Boolean functions of the form \(f(x)=tr_1^n(\lambda x^{2^{2r}+2^{r+1}+1}),\) \(\lambda\in\mathbb{F}_{2^n}\setminus\{0\}\), for n = 3r and n = 5r by investigating the lower bounds of the first order nonlinearity of their derivatives.
This is a preview of subscription content, log in via an institution to check access.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Preview
Unable to display preview. Download preview PDF.
Similar content being viewed by others
References
Canteaut, A., Charpin, P., Kyureghyan, G.M.: A New Class of Monomial Bent Functions. Finite Fields and Appli. 14, 221–241 (2008)
Carlet, C.: Recursive Lower Bounds on the Nonlinearity Profile of Boolean Functions and Their Applications. IEEE Trans. Inform. Theory 54(3), 1262–1272 (2008)
Carlet, C., Mesnager, S.: Improving the Upper Bounds on the Covering Radii of Binary Reed-Muller Codes. IEEE Trans. Inform. Theory 53(1), 162–173 (2007)
Courtois, N.T.: Higher Order Correlation Attacks, XL Algorithm and Cryptanalysis of Toyocrypt. In: Lee, P.J., Lim, C.H. (eds.) ICISC 2002. LNCS, vol. 2587, pp. 182–199. Springer, Heidelberg (2003)
Fourquet, R., Tavernier, C.: An Improved List Decoding Algorithm for the Second Order Reed-Muller Codes and its Applications. Designs Codes and Crypto. 49, 323–340 (2008)
Gangopadhyay, S., Sarkar, S., Telang, R.: On the Lower Bounds of the Second Order Nonlinearities of Some Boolean Functions. Information Sciences 180, 266–273 (2010)
Gangopadhyay, S., Singh, B.K.: On Second-Order Nonlinearities of Some Type Bent Functions, http://eprint.iacr.org/2010/286.pdf
Gode, R., Gangopadhyay, S.: On Lower Bounds of Second-Order Nonlinearities of Cubic Bent Functions Constructed by Concatenating Gold Functions. Int. J. Comput. Math. 88(15), 3125–3135 (2011)
Golić, J.: Fast Low Order Approximation of Cryptographic Functions. In: Maurer, U.M. (ed.) EUROCRYPT 1996. LNCS, vol. 1070, pp. 268–282. Springer, Heidelberg (1996)
Iwata, T., Kurosawa, K.: Probabilistic Higher Order Differential Attack and Higher Order Bent Functions. In: Lam, K.-Y., Okamoto, E., Xing, C. (eds.) ASIACRYPT 1999. LNCS, vol. 1716, pp. 62–74. Springer, Heidelberg (1999)
Kolokotronis, N., Limniotis, K.: Maiorana-McFarland Functions with High Second Order Nonlinearity, http://eprint.iacr.org/2011/212.pdf
MacWilliams, F.J., Sloane, N.J.A.: The Theory of Error Correcting Codes. North-Holland, Amsterdam (1977)
Rothaus, O.S.: On ”Bent” Functions. Journal of Combinatorial Theory, Series A 20, 300–305 (1976)
Sun, G., Wu, C.: The Lower Bounds on the Second-Order Nonlinearity of Three Classes of Boolean Functions with High Nonlinearity. Information Sciences 179(3), 267–278 (2009)
Sun, G., Wu, C.: The Lower Bounds on the Second-Order Nonlinearity of a Class of Boolean Functions with High Nonlinearity. Appli. Alg. in Eng. Commu. and Comp. 22, 37–45 (2011)
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2013 ICST Institute for Computer Science, Social Informatics and Telecommunications Engineering
About this paper
Cite this paper
Singh, D., Bhaintwal, M. (2013). On Second-Order Nonlinearities of Two Classes of Cubic Boolean Functions. In: Singh, K., Awasthi, A.K. (eds) Quality, Reliability, Security and Robustness in Heterogeneous Networks. QShine 2013. Lecture Notes of the Institute for Computer Sciences, Social Informatics and Telecommunications Engineering, vol 115. Springer, Berlin, Heidelberg. https://doi.org/10.1007/978-3-642-37949-9_49
Download citation
DOI: https://doi.org/10.1007/978-3-642-37949-9_49
Publisher Name: Springer, Berlin, Heidelberg
Print ISBN: 978-3-642-37948-2
Online ISBN: 978-3-642-37949-9
eBook Packages: Computer ScienceComputer Science (R0)