Skip to main page content
U.S. flag

An official website of the United States government

Dot gov

The .gov means it’s official.
Federal government websites often end in .gov or .mil. Before sharing sensitive information, make sure you’re on a federal government site.

Https

The site is secure.
The https:// ensures that you are connecting to the official website and that any information you provide is encrypted and transmitted securely.

Access keys NCBI Homepage MyNCBI Homepage Main Content Main Navigation
. 2019 Apr 9;9(1):5801.
doi: 10.1038/s41598-019-42243-5.

A Computational Design Framework for Efficient, Fabrication Error-Tolerant, Planar THz Diffractive Optical Elements

Sourangsu Banerji  1 , Berardi Sensale-Rodriguez  2
Affiliations

A Computational Design Framework for Efficient, Fabrication Error-Tolerant, Planar THz Diffractive Optical Elements

Sourangsu Banerji et al. Sci Rep. .

Abstract

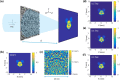
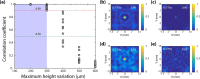
We demonstrate ultra-thin (1.5-3λ0), fabrication-error tolerant efficient diffractive terahertz (THz) optical elements designed using a computer-aided optimization-based search algorithm. The basic operation of these components is modeled using scalar diffraction of electromagnetic waves through a pixelated multi-level 3D-printed polymer structure. Through the proposed design framework, we demonstrate the design of various ultrathin planar THz optical elements, namely (i) a high Numerical Aperture (N.A.), broadband aberration rectified spherical lens (0.1 THz-0.3 THz), (ii) a spectral splitter (0.3 THz-0.6 THz) and (iii) an on-axis broadband transmissive hologram (0.3 THz-0.5 THz). Such an all-dielectric computational design-based approach is advantageous against metallic or dielectric metasurfaces from the perspective that it incorporates all the inherent structural advantages associated with a scalar diffraction based approach, such as (i) ease of modeling, (ii) substrate-less facile manufacturing, (iii) planar geometry, (iv) high efficiency along with (v) broadband operation, (vi) area scalability and (vii) fabrication error-tolerance. With scalability and error tolerance being two major bottlenecks of previous design strategies. This work is therefore, a significant step towards the design of THz optical elements by bridging the gap between structural and computational design i.e. through a hybrid design-based approach enabling considerably less computational resources than the previous state of the art. Furthermore, the approach used herein can be expanded to a myriad of optical elements at any wavelength regime.

PubMed Disclaimer

Conflict of interest statement

The authors declare no competing interests.

Figures

Figure 1
Figure 1
(a) Flow diagram of the Gradient Descent Assisted Binary Search (GDABS) algorithm. The right panels depict convergence plots of efficiency with number of iterations for (b) high N.A. broadband aberration rectified spherical lens with a two-fold symmetry (i.e. left to right flip symmetry and rotational symmetry) condition, (c) spectral splitter with no symmetry condition and (d) an on-axis broadband transmissive hologram with one-fold symmetry (i.e. left to right flip symmetry) condition.
Figure 2
Figure 2
(a) Schematic of the high N.A. broadband aberration rectified spherical lens with a focal length f = 10 mm under broadband illumination (λ1 = 3 mm [0.1 THz] and λ2 = 1 mm [0.3 THz]). (b) Pixel height distribution for the spherical lens with a maximum height of 3.5 mm. The dimensions (length and width) of the designed lens was 26 mm. The pixelation is along both x and y directions. (c) Optical image of a fabricated spherical lens using a common 3D printer. PLA was the material used to print the structure. The bottom left panels depict the (d,e) scalar predication based semi-analytic and (f,g) measured on-axis point spread functions (PSFs) for 0.1 THz and 0.3 THz. The bottom right panels depict the (h,i) z-propagation scalar plot for the designed spherical lens.
Figure 3
Figure 3
(a) Schematic of the spectral splitter with a splitting distance d = 50 mm under broadband illumination (λ1 = 0.75 mm [0.4 THz], λ2 = 0.6 mm [0.5 THz], and λ3 = 0.5 mm [0.6 THz]). The designed structure splits the incoming THz wave into a series of spatially separated lines at the pre-setted designed distance. (b–e) Pixel height distribution for the spectral splitter with a maximum pixel height of 2 mm. The dimensions (length and width) of the spectral spiltter was 52 mm. The pixelation is only along x direction. The bottom panels depict the designed spatial profile for splitter elements with uniform splitting at an inter-spatial distance of (f) 20 mm, and (g) 10 mm and also spectral splitters with an arbitrary splitting at a inter-spatial distance of (h) 20 mm, and (i) 10 mm. The insets give the corresponding on-axis PSFs plots for the respective THz frequencies.
Figure 4
Figure 4
(a) Schematic of an on-axis broadband transmissive hologram with a operating distance d = 50 mm under broadband illumination (λ1 = 0.75 mm [0.4 THz], λ2 = 0.6 mm [0.5 THz], and λ3 = 0.5 mm [0.6 THz]). (b) The _target image which was used during the optimization. (c) Pixel height distribution for the hologram with a maximum pixel height of 2.5 mm. The dimensions (length and width) of the hologram was 26 mm. The pixelation is along both x and y directions. The left panels depict the scalar predication based semi-analytic on-axis point spread functions (PSFs) for (d) 0.3 THz, (e) 0.4 THz and (f) 0.5 THz.
Figure 5
Figure 5
(a) Robustness of the designs to fabrication (standard 3D printing) errors. Random height variations of maximum value ΔE are introduced in the designs. Correlation coefficient is then computed between the diffraction patterns of the original and modified designs. The diffraction patterns are calculated on basis of the scalar predication based semi-analytic model. The correlation coefficient is then plotted versus ΔE for the designed lenses. The right panels depict the PSFs corresponding to 0.1 THz and 0.3 THz for (b,c) 0.90 correlation coefficient and (d,e) 0.50 correlation coefficient.
See this image and copyright information in PMC

Similar articles

See all similar articles

Cited by

References

    1. Goodman, J. W. Introduction to Fourier Optics (2005).
    1. Lalanne P, Chavel. P. Metalenses at visible wavelengths: past, present, perspectives. Las. & Phot. Rev. 2017;11:1600295. doi: 10.1002/lpor.201600295. - DOI
    1. Lewis, R. A. Terahertz Physics (2012).
    1. Tonouchi M. Cutting-edge terahertz technology. Nat. Phot. 2007;1:97.
    1. Hu BB, Nuss MC. Imaging with terahertz waves. Opt. Lett. 1995;20:1716–1718. doi: 10.1364/OL.20.001716. - DOI - PubMed
  NODES
3d 4
coding 1
HOME 2
Javascript 1
os 6
text 12
twitter 2
web 4